Resonance: When a body capable of vibration is displaced and then allowed to vibrate freely, it will vibrate with a frequency which is called the natural frequency. If an external periodic force is applied to the body and the body vibrates with the frequency of the force, the motion is called forced vibration. In forced vibration, if the frequency of the periodic force is equal to the natural frequency of the body, the amplitude of the vibration becomes very large and the vibration of the body is called resonance.

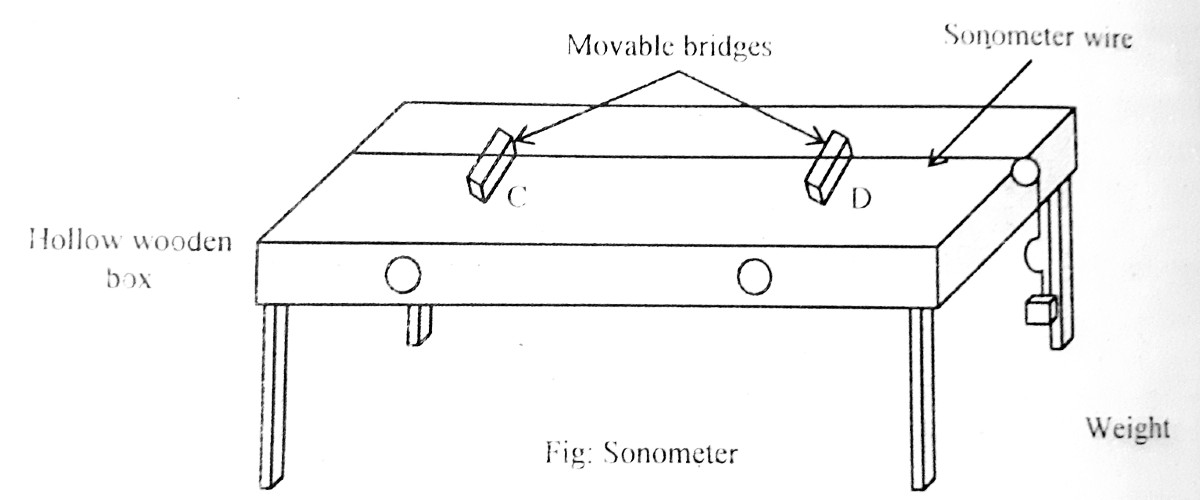
A sonometer consists essentially of a thin metallic wire stretched across two bridges A and B on the top of a hollow, wooden sounding box about 1 meter long. One end of the wire is fastened to a peg at one end of a box. Its other end passes over a smooth frictionless pulley fixed at the other end of the box and carries a scale pan so that it can be loaded to have any desired tension. There are two bridges C and D between the two fixed bridges A and B sliding over a scale to adjust the length of the vibrating position of the string.

To obtain the frequency of the tuning fork, take a resonance vibration of the string, a small 'V' shaped piece of paper is placed on the string between bridges C and D. Some weight (W) is put on the scale pan to produce tension in the string. A tuning fork is stuck against a rubber pad and placed in the box close to the segment CD of the wire. When the string between the bridges vibrates, the piece of paper on it flutters. by varying the resonating length of the string by moving C or D, if the frequency of vibration of the string given by the equation, \(f=\frac1{2l}\sqrt{\frac T\mu}\) becomes equal to the frequency of the tuning fork, the string segment CD vibrates with the maximum amplitude. As a result, the piece of paper may fall. This is how the resonant condition is detected. at this condition, the resonant length and weight on the pan are noted. By knowing the value of mass per unit length of the wire we can calculate the frequency of the tuning fork.